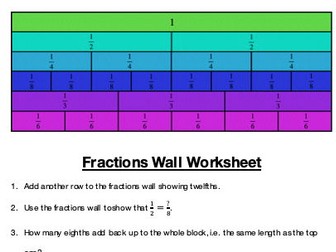
Fractions (Blocks/Wall/Pictorial) Lessons And Worksheet
<p>This pack contains three ways to approach fractions and a worksheet on fractions walls. The first is a basic approach suited to students seeing the topic for the first time or recapping after a first lesson (Pathway 1). It does not introduce the concept by splitting a single object into parts and emphasises sharing collections between people, after some of my primary school students struggled to transition from visualising cakes/pizzas to tackling fractions of numbers.</p>
<p>The second is a refresher for older students, using the traditional depiction with circles/pizzas and fraction walls (Pathway 2).</p>
<p>The third is an alternative approach to solving more complex fraction problems by visualising it with blocks. This was a massive help for some of my GCSE learners who were struggling with percentages - the lesson begins by introducing the blocks for use with fractions and can then be continued to make fractions and percentages identical.</p>