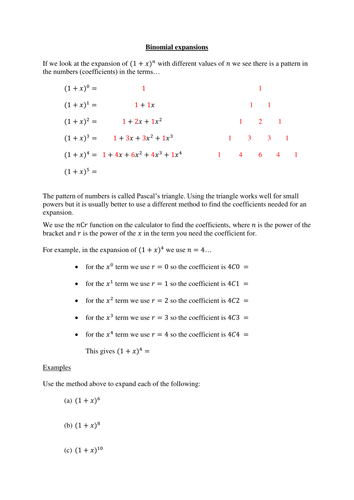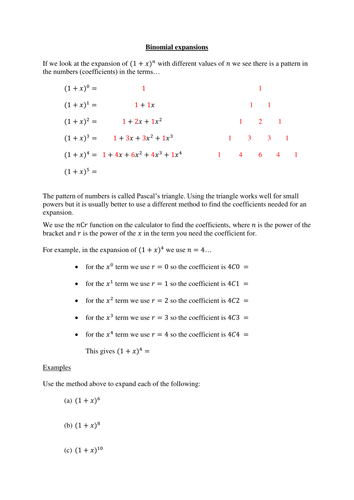
This resource can be used to quickly introduce the method for expanding expressions of the form (1+ax)^n where n is a positive integer.
It begins by showing expansions of (1+x)^n for small values of n and highlights the coefficients to introduce Pascal's triangle. It then shows how nCr can be used to find the required coefficients in the expansions and has a few expansions of the form (1+x)^n for students to complete.
Next is a worked example expanding (1-x)^n to introduce the technique and the pattern of the signs of the terms in the expansion, followed by a few expansions of the form (1-x)^n for students to complete.
Next is a worked example expanding (1+ax)^n to introduce the technique and the best way to set out the working, followed by a few expansions of the form (1+ax)^n for students to complete.
The answers to all the expansions are included.
It begins by showing expansions of (1+x)^n for small values of n and highlights the coefficients to introduce Pascal's triangle. It then shows how nCr can be used to find the required coefficients in the expansions and has a few expansions of the form (1+x)^n for students to complete.
Next is a worked example expanding (1-x)^n to introduce the technique and the pattern of the signs of the terms in the expansion, followed by a few expansions of the form (1-x)^n for students to complete.
Next is a worked example expanding (1+ax)^n to introduce the technique and the best way to set out the working, followed by a few expansions of the form (1+ax)^n for students to complete.
The answers to all the expansions are included.
Something went wrong, please try again later.
This resource hasn't been reviewed yet
To ensure quality for our reviews, only customers who have downloaded this resource can review it
Report this resourceto let us know if it violates our terms and conditions.
Our customer service team will review your report and will be in touch.
£0.00