Confidence Interval Estimation (Statistics)

Confidence Interval Estimation is a lecture which is covered within the Statistic or Basic Business Statistic module by business and economics students.

Suppose you want to estimate the mean GPA of all the students at your university. The mean GPA for all the students is an unknown population mean, denoted by u. You select a sample of students and compute the sample mean, denoted by X, to be 2.80. As a point estimate of the population mean, u, you ask how accurate is the 2.80 value as an estimate of the population mean, u? By considering the variability from sample to sample (see Section 7.2, concerning the sampling distribution of the mean), you can construct a confidence interval estimate for the population mean to answer this question.

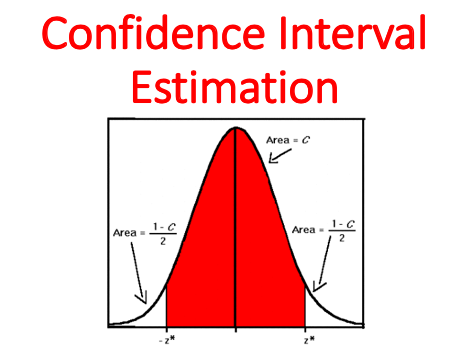
When you construct a confidence interval estimate, you indicate the confidence of correctly estimating the value of the population parameter, u. This allows you to say that there is a specified confidence that u is somewhere in the range of numbers defined by the interval. After studying this chapter, you might find that a 95% confidence interval for the mean GPA at your university is 2.75 < u < 2.85. You can interpret this interval estimate by stating that you are 95% confident that the mean GPA at your university is between 2.75 and 2.85.

In this chapter, you learn to construct a confidence interval for both the population mean and population proportion. You also learn how to determine the sample size that is necessary to construct a confidence interval of a desired width.

In this lecture, you learn to:

1. To construct and interpret confidence interval estimates for the population mean and the population proportion
2. To determine the sample size necessary to develop a confidence interval for the population mean or population proportion
3. How to use confidence interval estimates in auditing
4. When to use a finite population correction factor in calculating a confidence interval for either µ or π
5. How to use a finite population correction factor in calculating a confidence interval for either µ or π
6. How to use a finite population correction factor in calculating a sample size for a confidence interval for either µ or π
7. The concept of bootstrapping and when it makes sense to use it.

In this file you will find:

• Confidence Interval Estimation Lecture Power Point Presentation
• Confidence Interval Estimation Test Bank with 183 different related questions with full answer description and explanation
• 68 Exercises related to the topic with all answers to them
• Confidence Interval Estimation Reading Resources file in order to enhance Lecturer/Teacher/Student knowledge

Once you will purchase this resource please leave a comment!
All resources are compressed in zip file.
You can purchase this teaching resource with more than 20 % Discount by pressing this link. Use counpon code during checkout process: LOVETOTEACH