
A complete lesson on the graph of tangent from 0 to 360 degrees. I’ve also made complete lessons on sine and cosine from 0 to 360 degrees and all three functions outside the range 0 to 360 degrees.
Designed to come after pupils have been taught about the ratios sine, cosine and tangent in the context of right-angled triangle trigonometry, and have met the unit circle definitions of sine and cosine.
Activities included:
Starter:
A quick set of questions on finding the gradient of a line. This is a prerequisite to understanding how tan varies for different angles.
Main:
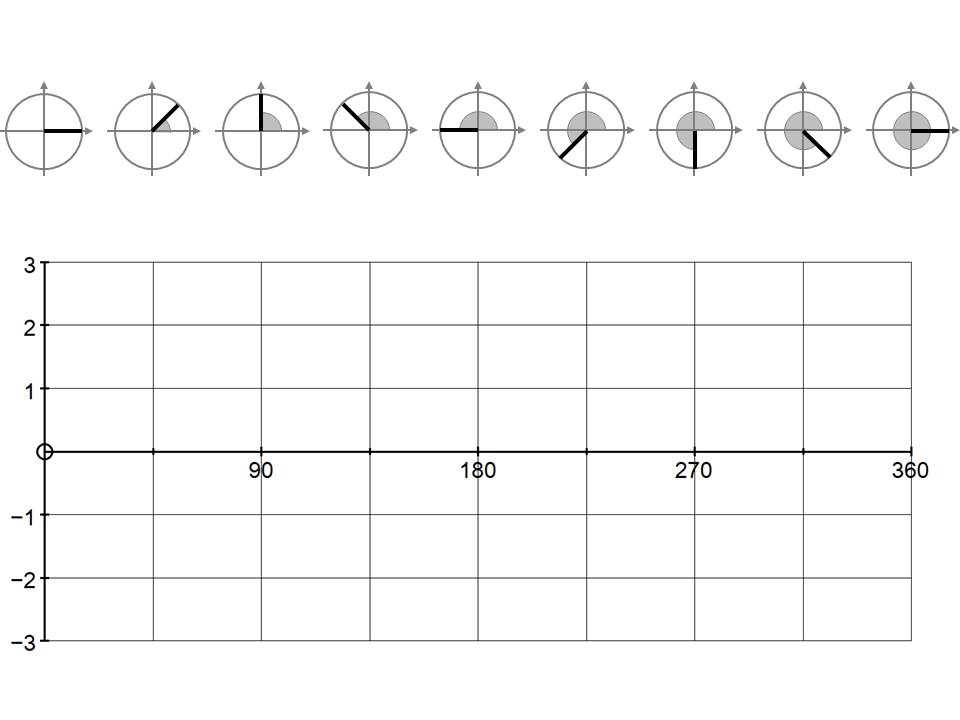
- An example to remind pupils how to find an unknown angle in a right-angled triangle using the tangent ratio, followed by a set of similar questions. The intention is that pupils estimate using the graph of tangent rather than using the inverse tan key on a calculator, to refamiliarise them with the graph from 0 to 90 degrees.
- Slides to define tan as sin/cos and hence as gradient when using the unit circle definition. A worksheet where pupils construct the graph of tan from 0 to 360 degrees (see cover image).
-
- A set of related questions, where pupils use graph and unit circle representations to explain why pairs of angles have the same tan. Pupils can be extended further by making and proving conjectures about pairs of angles whose tans are equal.
Plenary:
An image to prompt discussion about the “usual” definition of tangent (using the terminology opposite, adjacent and hypotenuse) and the fuller definition (using the unit circle)
Printable worksheets and answers included.
Please review if you buy as any feedback is appreciated!
Something went wrong, please try again later.
This resource hasn't been reviewed yet
To ensure quality for our reviews, only customers who have purchased this resource can review it
Report this resourceto let us know if it violates our terms and conditions.
Our customer service team will review your report and will be in touch.