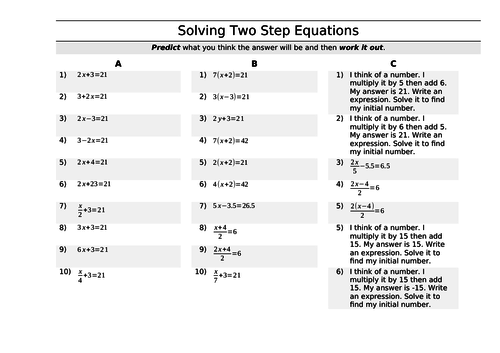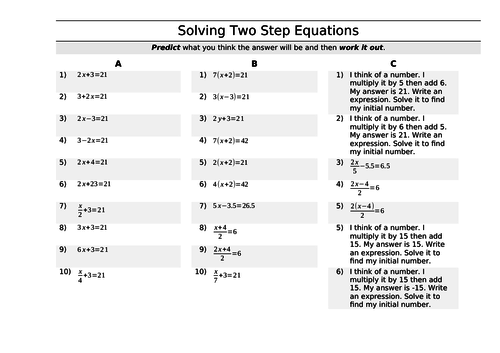
Trying to use variation theory
My thinking
- A question to start
- Reversing the terms. Does balancing still work?
- A subtraction. How does this effect our balance.
- Does reversing the terms still lead us to the same answer
- Increasing the constant by one. What happens? Also: a decimal answer.
- We can have a negative answer
- Divide x, instead of multiplying it.
- Increasing co-efficient of x by one. What happens to our answer?
- Doubling co-efficient of x. Not sure about these last two. I think they may be a step back from question 7. This is the problem with presenting these in a linear format. These questions are variations on question 1, not question 7. I might experiment with some kind of spider diagram.
- Doubling the divisor from 7. Again, maybe the linear way these are written is a bit rubbish.
Don’t know how I like the order of these questions, but there’s lots to think about and something to tweak.
I have found the transition to asking ‘why have they asked you that question? What are they trying to tell you?’ has been difficult for some students, but I think it’s worth devoting time to it. If students are inspecting questions for things like this, maybe they’re more likely to read the question thoroughly and pick out it’s mathematics. Big hope, I know.
Something went wrong, please try again later.
I like the idea of asking students why they have been asked a certain question. I t requires them to think "why" and that's half the battle. Thank you for sharing.
A handy set of equation-solving questions, grouped by difficulty. Thanks for sharing.
Report this resourceto let us know if it violates our terms and conditions.
Our customer service team will review your report and will be in touch.