The first resource introduces the technique for writing a complex number z=a+bi in (trigonometric) polar form, r(cos (theta)+ i sin(theta)), there are few examples of converting from one form into the other (to do as a class), and then an exercise of 30 questions for students to do. The next section introduces the exponential polar form re^(i theta), a few examples of converting from one form into the other (to do as a class), and then an exercise of questions for students to do. The exercise includes questions that get students to consider what z* and -z look like in both polar forms, as well as investigating multiplying and dividing complex numbers in polar form. Answers to the exercises are included.
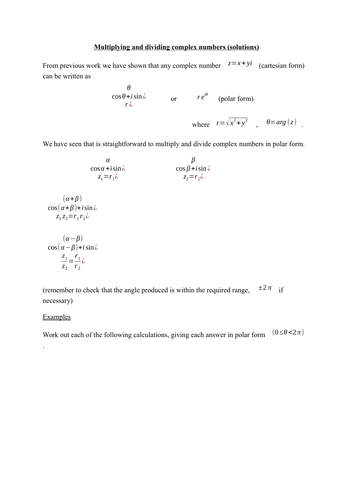
The second resource begins with a reminder of how to multiply/divide complex numbers in polar form, followed by an exercise of questions to practise. The remaining 3 pages cover the geometrical effect of multiplying, with several examples for students to learn from. Fully worked solutions are included.
The final resource focuses on examination-style questions that consider the geometric effect of multiplying by a complex number in polar form. Fully worked solutions are included.
The second resource begins with a reminder of how to multiply/divide complex numbers in polar form, followed by an exercise of questions to practise. The remaining 3 pages cover the geometrical effect of multiplying, with several examples for students to learn from. Fully worked solutions are included.
The final resource focuses on examination-style questions that consider the geometric effect of multiplying by a complex number in polar form. Fully worked solutions are included.
Something went wrong, please try again later.
This resource hasn't been reviewed yet
To ensure quality for our reviews, only customers who have purchased this resource can review it
Report this resourceto let us know if it violates our terms and conditions.
Our customer service team will review your report and will be in touch.
£3.00