Second order differential equations worksheet

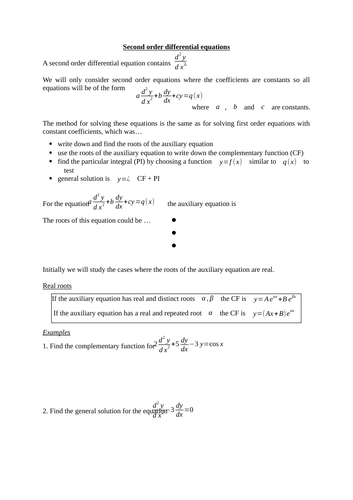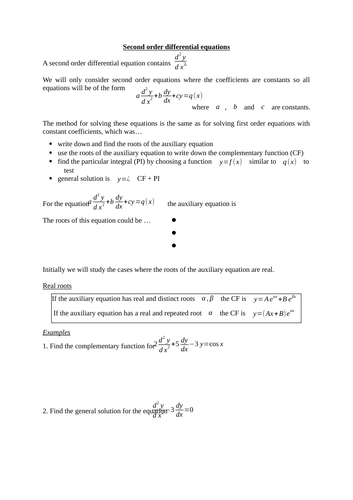
This 12 page resource covers the solution of 2nd order differential equations by finding the roots of its auxiliary equation, and its particular integral.

The first section focuses on cases where the auxiliary equation has real roots (distinct or repeated). It begins by concentrating on finding only the complementary function - there are several examples to work through with your class and then an exercise with 14 questions for students to attempt. There are then a few examples that involve finding both the complementary function and the particular integral.

The second section focuses on cases where the auxiliary equation has complex roots (a+/-bi or +/-bi). There are several examples to work through with your class and then an exercise with 18 questions for students to attempt. The exercise includes questions where students are required to consider the behaviour of the solution (bounded/unbounded oscillations) when x becomes large, as well as the function to which the solution approximates when x becomes large.

Answers to both exercises are included.