A.1.3.4 Classification by temperature, black body radiation
Stefan’s law and Wien’s displacement law.
General shape of black body curves, experimental verification is not required. Use of Wien’s displacement law to estimate black-body temperature of sources λmaxT = constant = 2.9 × 10⁻³mK. Inverse square law, assumptions in its application. Use of Stefan’s law to estimate area needed for sources to have same power output as the sun. P = σAT⁴ Assumption that a star is a black body.
A.1.3.5 Principles of the use of stellar spectral classes
Temperature related to absorption spectra limited to Hydrogen Balmer absorption lines: need for atoms in n = 2 state.
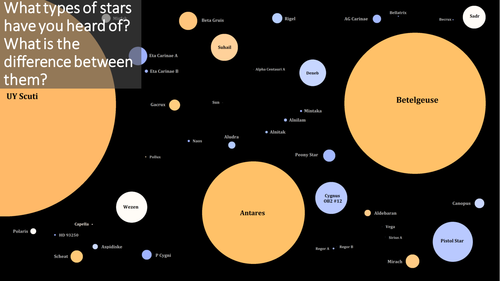
A.1.3.6 The Hertzsprung-Russell diagram
General shape: main sequence, dwarfs and giants.
Axis scales range from –15 to 10 (absolute magnitude) and 50 000K to 2 500K (temperature) or OBAFGKM (spectral class).
Stellar evolution: path of a star similar to our Sun on the Hertzsprung-Russell diagram from formation to white dwarf.
A.1.3.7 Supernovae, neutron stars and black holes
Defining properties: rapid increase in absolute magnitude of supernovae; composition and density of neutron stars; escape velocity > c for black holes.
Use of supernovae as standard candles to determine distances. Controversy concerning accelerating Universe and dark energy.
Supermassive black holes at the centre of galaxies.
Calculation of the radius of the event horizon for a black hole
Schwarzschild radius ( Rs )
Stefan’s law and Wien’s displacement law.
General shape of black body curves, experimental verification is not required. Use of Wien’s displacement law to estimate black-body temperature of sources λmaxT = constant = 2.9 × 10⁻³mK. Inverse square law, assumptions in its application. Use of Stefan’s law to estimate area needed for sources to have same power output as the sun. P = σAT⁴ Assumption that a star is a black body.
A.1.3.5 Principles of the use of stellar spectral classes
Temperature related to absorption spectra limited to Hydrogen Balmer absorption lines: need for atoms in n = 2 state.
A.1.3.6 The Hertzsprung-Russell diagram
General shape: main sequence, dwarfs and giants.
Axis scales range from –15 to 10 (absolute magnitude) and 50 000K to 2 500K (temperature) or OBAFGKM (spectral class).
Stellar evolution: path of a star similar to our Sun on the Hertzsprung-Russell diagram from formation to white dwarf.
A.1.3.7 Supernovae, neutron stars and black holes
Defining properties: rapid increase in absolute magnitude of supernovae; composition and density of neutron stars; escape velocity > c for black holes.
Use of supernovae as standard candles to determine distances. Controversy concerning accelerating Universe and dark energy.
Supermassive black holes at the centre of galaxies.
Calculation of the radius of the event horizon for a black hole
Schwarzschild radius ( Rs )
Something went wrong, please try again later.
Fantastic - great quality
thank you for sharing
Very helpful ! Thank you
Really helpful ,very clear Thankyou
Report this resourceto let us know if it violates our terms and conditions.
Our customer service team will review your report and will be in touch.
£0.00