
Something went wrong, please try again later.
Thank you for publishing your resource. It has been selected to be featured in <a href="https://www.tes.com/teaching-resources/collections/secondary-maths/"> a new secondary maths collection</a>.
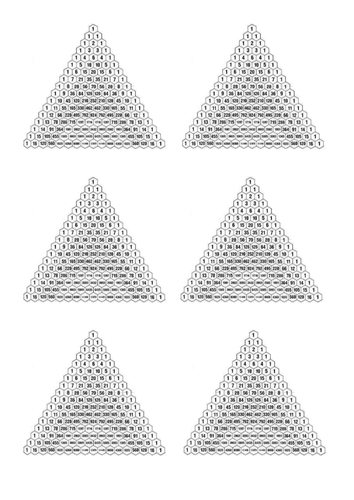
If you fancy a task that is a little bit different, then look no further than one of Mr Barton Maths rich tasks. This task in particular looks at Pascal's triangle, how it is formed and what patterns students can see within the triangles when they are asked to shade in different multiples. A perfect way to highlight that sequences isn't just sets of numbers.
As always, a great (set of) resource(s).
• Describe how Pascal’s triangle has been formed<br /> • What patterns can you discover?<br /> • What patterns do shading the multiples of 2, 3, 4, etc make?<br /> • Investigate the diagonals<br /> • Investigate the sums of the horizontal rows<br /> • Shade odd and even numbers<br /> • Can you see the square numbers anywhere?<br /> • Can you see the Fibonacci sequence anywhere?<br /> • Can you see the powers of 11 anywhere?<br /> • Can you see a link to the probability of tossing coins?<br /> • Try investigating “hockey stick” patterns<br /> • What other triangles can you create by changing the rules?<br />
Report this resourceto let us know if it violates our terms and conditions.
Our customer service team will review your report and will be in touch.
