Vary and Twist: Simplifying Ratio

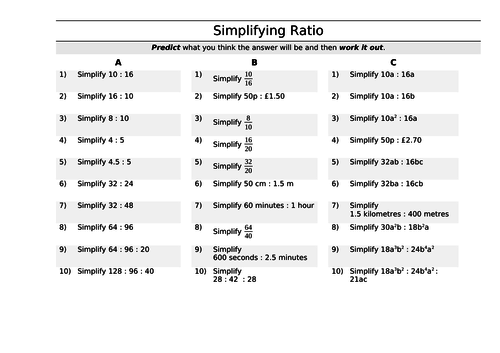
An attempt at some variation theory

This one was hard. I spent ages rearranging questions and looking at what should be added. Specifically, I had a massive dilemma when it came to introducing fractions. I was trying to point out the ways in which simplifying fractions and simplifying ratio were similar, but I’m not sure that I haven’t just led students down the wrong path thinking they’re equivalent. For instance 5 : 6 is 5/11 and 6/11, not 5/6. Hmmmm.

The variations I used for section A.

1. An example where you can use a prime divisor
2. The opposite way around. What happens to our answer. Order is important!
3. Half one side. 8 : 5 becomes 4 : 5
4. One that’s already as simple as possible. Time for some questioning? How do you know you can’t simplify it?
5. It’s not just reducing the numbers down. Here you have to multiply up. Deals with what simple is. I have changed this from the picture to make only one number vary from the previous question.
6. Needs a non prime divisor. This isn’t really a variation, though. It has nothing really to do with the previous questions!
7. Again, double one side
8. Double both. Our answer does not double!
9. Adding a third part of the ratio. Changes the answer significantly.
10. Doubling two parts here. Our parts don’t double in our answer!

If you amend this and it works better, please let me know.