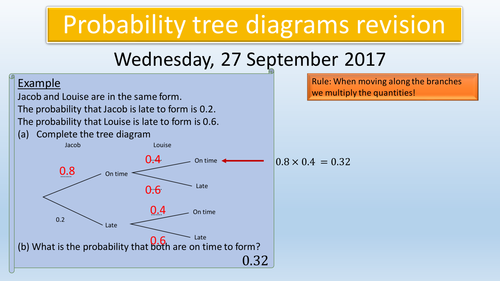
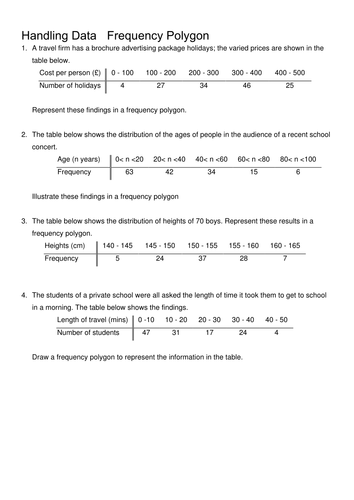
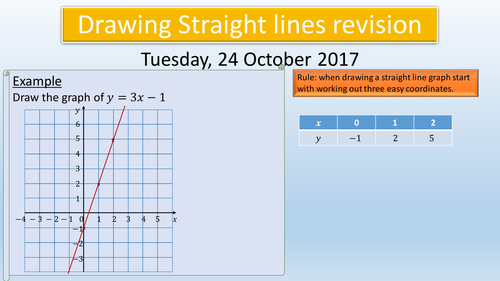
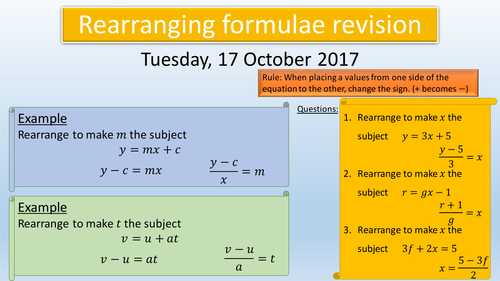
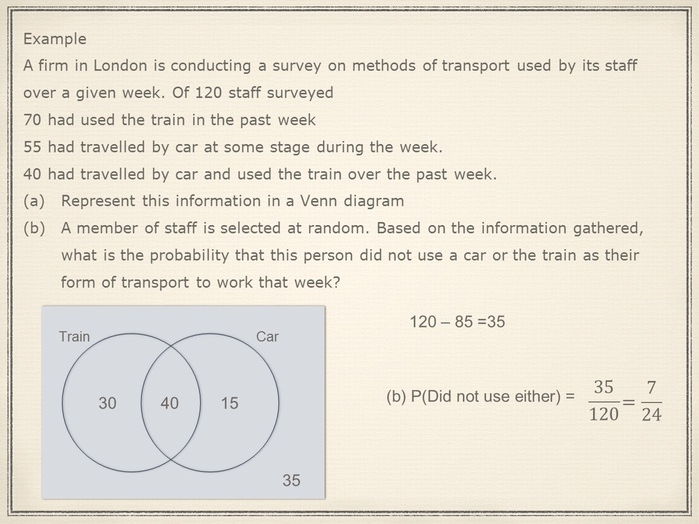
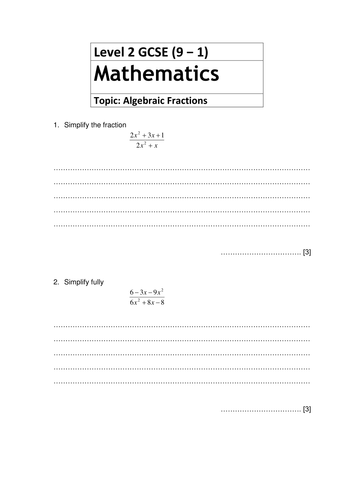
ReallyUsefulMaths
The Resources within this shop are all designed for the teaching of Mathematics for those in the age range 7 - 18 years old. Most resources consist of a PowerPoint lesson followed by a worksheet for the students. With over twenty nine years of experience, the powerpoint/worksheets within the shop have been used successfully by myself and colleagues over that time. As a head of department for over 15 years, the department has yearly been judged as adding substantial value to students grades.