Factors Multiples Primes: Teaching for Mastery in Primary Maths ǀ Tes

Progression in learning explained by topic

Number and place value Addition and subtraction Multiplication and division Fractions Length, weight, area and volume Time Geometry Statistics Factors, multiples, primes Negative numbers Calculations with four operations Ratio and proportion Algebra Roman numerals Decimals Percentages Money Times tables and skip counting

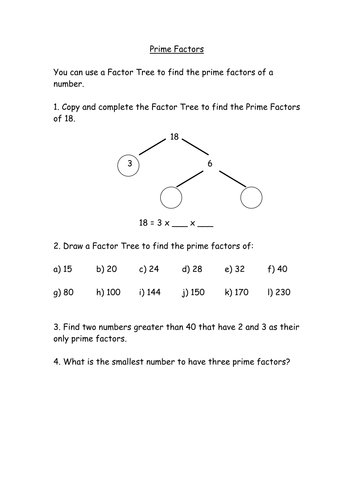
Number: Factors, multiples and primes

The concepts explored in this chapter underpin many techniques and proofs in mathematics and are the basis of an extensive area of academic research known as number theory.

This topic will likely be studied alongside others on number properties, multiplication and division, as they are so closely intertwined. In fact, these concepts have connections across the majority of topic areas. Lowest common multiples, for example, crop up when adding fractions and factors playing a vital role in tessellation.

Broadly speaking, pupils will learn that if one number goes into another number evenly, then it is a factor of the number it goes into. For example, 5 goes into 10 evenly (ie, without a remainder), so 5 is a factor of 10. Similarly, as 2 goes into 10 evenly, 2 is also a factor of 10.

Pupils will also see that multiples are the opposite of factors and will understand that if you multiply a number by an integer, then the new number is a multiple of the first. For example, 10 × 7 = 70, so 70 is a multiple of 10.

Factors, multiples and primes: Teaching for mastery booklet

Brought to you by Tes in partnership with experts at Mathematics Mastery and the White Rose Maths Hub, this PDF provides teachers with the progression of learning for the topic, broken down into digestible chapters.

It is an ideal starting point for primary teachers looking to brush up on their subject knowledge, or adopt the mastery approach to maths in their classroom.

Check out the Teaching for mastery site, which contains schemes of work accompanied by quality-assured classroom resources.

Download resources to match this topic in the planning and lesson resources pages of the new primary maths section.

By TES Resource Team

Teacher's guide: Teaching for mastery booklet

It is an ideal starting point for primary teachers looking to brush up on their subject knowledge, or adopt the mastery approach to maths in their classroom.

Check out the Teaching for mastery site, which contains schemes of work accompanied by quality-assured classroom resources.

Download resources for all primary maths topics in the planning and lesson resources pages of the new primary maths section.

By TES Resource Team

Chapter 1: Factor pairs

In this chapter, pupils use their knowledge of multiplication to recognise and use factor pairs.

Building on their knowledge of the commutative property of multiplication (ie, that 6 x 4 = 4 x 6), pupils realise that the order of a factor pair does not alter the product. With this in mind, they use factor pairs in calculations. For example, in calculating 16 x 25, pupils recognise that 16 = 4 x 4 and find 4 x 25 x 4 = 100 x 4 = 400.

While this topic deserves dedicated lesson time, it is important that opportunities to use factor pairs and commutativity are planned for throughout the year. Additionally, these concepts should be referenced and applied across the rest of the mathematics curriculum.

Chapter 2: Factors, multiples and prime numbers

In this chapter, pupils learn about factors, multiples and prime numbers, as well as square and cube numbers.

In preparation for work with primes, pupils find all factor pairs of a number and identify the common factors of two numbers.

Following an introduction, pupils know that a number is considered a prime if it has no factor except 1 and itself. Using their knowledge of factors, they establish whether this is the case for any number up to 100. They also recall prime numbers up to 19 through frequent practice and application.

Later, pupils recognise and use square and cube numbers. They should experience concrete and pictorial representations of both number types in order to understand why they are described this way. Pupils are introduced to the notation for squared (²) and cubed (³) and solve problems involving square and cube numbers.

Chapter 3: Common factors and multiples

In this chapter, pupils identify common multiples and continue to identify common factors and prime numbers.

Pupils continue to work with prime numbers and, in doing so, use language such as “prime” and “composite”.

Additionally, they identify common factors and multiples and talk about them using vocabulary such as “factor”, “common factor”, “multiple” and “common multiple”. Pupils illustrate these concepts using objects and diagrams (eg, an array model). They also use their knowledge in other areas of the mathematics curriculum (eg, to find equal denominators when adding and subtracting fractions).

Approved resources

Prime Factors using a factor tree

This worksheet is for pupils who are learning about prime factorisation. Please give me some feedback on this, as I am a student teacher and would value all feedback.

By M Allan

Related resources

KS3/KS2 Maths Factor trees worksheet

Maths Worksheet for classwork or homework. Factor Trees. Objective: to be able to use factor trees to express numbers as products as their prime factors A worksheet for prime factor decomposition, starter covers primes, factors and products, main is making factor trees and writing the number as a product of prime factors. Extension asks students to try to spot link between prime factors and HCF and LCM

By bcooper87

Products and Factors Investigations

Investigations involving products and factors for higher ability maths group.

By Miss Britton