- Home
- Why every primary should be using bar modelling - and six steps to make it a success
Why every primary should be using bar modelling - and six steps to make it a success

As a primary maths coordinator, it’s been difficult to escape the lure of bar modelling: it’s in every new publication, on all the maths blogs and at every coordinator’s meeting. And so, when the time was right for my school, I succumbed.
Bar modelling, for the uninitiated, is not a method of calculation. Instead, it is a way of representing problems pictorially: from simple addition, through to finding percentages of amounts, all the way to complex multi-step problems involving ratio and proportion. Bar models can be used to pictorially represent arithmetic problems, as well as reasoning problems written with a context.
The reading comprehension involved in problem-solving hampers our children, and while bar modelling doesn’t remove the necessity to read and comprehend maths problems, it certainly provides a structured way of working through a written problem.
A worked example of bar modelling
Take this problem for example:
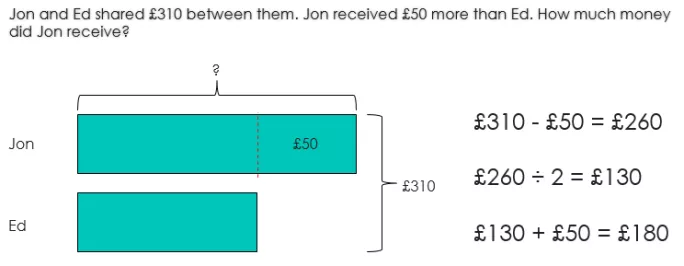
Jon and Ed shared £310 between them. Jon received £50 more than Ed. How much money did Jon receive?
Here we use a comparison model with two bars that represent Jon and Ed’s money. We then follow this process:
- We know that Jon has more than Ed, so his bar is longer.
- Using a bracket we show that together the two bars represent the total: £310.
- We prompt children to use the question mark (with a bracket in this case) to represent missing information: Jon’s total.
- Then we can label the part of Jon’s bar that is longer than Ed’s as his extra £50.
- Now children see that Jon has the same amount as Ed, plus £50.
At this point, the model has done its job, and it’s over to the calculations:
- We subtract £50 from £310, leaving £260.
- This £260 is split into two equal amounts as represented by the model; we divide £260 by 2 to get £130.
- Children may want to edit the bar model to show that Ed’s amount is £130 and that the first part of Jon’s bar (equal to Ed’s bar) is also worth £130.
- To find out how much Jon has we add £130 to £50 to get the final answer of £180.
Getting started
Inspired by a White Rose Maths Hub training session and Nick Hart’s blog post ”Throwing Out That Old RUCSAC” I set about introducing bar modelling to key Stages 1 and 2 of my school, mainly in order to combat the issues children face when solving worded problems. After a whole Friday of leading our school’s training I received the greatest compliment a CPD leader can get: teachers across the school were using it on Monday morning. They’d glimpsed the potential of bar modelling and couldn’t wait to try it out.
And, boy, has it been worth it. While some of our more able mathematicians struggled to see the point of it (they’re now accepting of the fact it does help them with more complex problems) we have seen children who have long struggled with maths suddenly experience absolute clarity on matters that previously confounded them. It’s given those children a greater level of independence and is helping them to achieve the same things as children considered as “more able”.
In short, bar modelling is removing barriers and opening up new possibilities to those who once found maths difficult. So what can I recommend to ensure it is successful in your school, too?
- Stick with it
Don’t give up after the first attempt. As with any new approach, it will take time to embed and you may face some resistance from children and even staff members (investing in training in order to get buy-in will alleviate this as much as possible).
- Practice makes permenant
Give children plenty of practice. The more they practise the method, the more it will become second nature and they will begin to “see” maths problems as bar models, thus helping them to visualise and better understand the inner workings of a problem.
- Guidance is crucial
Always encourage children to ask “Where does the question mark go?” This helps children to think through what information they already know and what information they are trying to find out. Often children will work out part of a problem, but not all of it - encouraging them to ask this question before they solve anything guides them towards completing the whole problem.
- Don’t be restrictive
Be creative - think of all possible applications, use it across a range of maths topics. Not only will this strengthen children’s understanding of bar modelling, it will also improve their understanding in other areas of maths as they will have a familiar way to visualise the problems.
- Start them young
Start bar modelling early. Bar models can be used very successfully at KS1. Start with part-whole bar models using pictures and move to discrete bar models where each square represents one. As the years go by, move children on to continuous part-whole bar models and comparison models, with a view to eventually removing the need for bar models altogether in most instances.
- Vocalise the process
Use them as a talking point and keep the vocabulary consistent. Talk about wholes, size of parts and number of parts. Talk about parts being either known or unknown. Use the models for partner work where children are verbalising the problem-solving process using the bar model as a prompt.
Aidan Severs is an assistant vice-principal at a primary school in the North of England
Want to keep up with the latest education news and opinion? Follow TES on Twitter and like TES on Facebook
Keep reading for just £1 per month
You've reached your limit of free articles this month. Subscribe for £1 per month for three months and get:
- Unlimited access to all Tes magazine content
- Exclusive subscriber-only stories
- Award-winning email newsletters



