Take a look at this Year 6 Sats question from last year’s reasoning paper.

What might your pupils do with it? Are some likely to go straight to calculating using formal long multiplication methods?

While formal methods have their place, they aren’t always the most efficient choice and developing fluency beyond them can support Sats success and improve pupils’ confidence in tackling both familiar and unfamiliar problems.

Using the distributive law, referred to throughout the national curriculum, helps make multiplication problems easier to solve by breaking them down into smaller multiplication facts, which are easier for pupils to recall.

Representations like the array (where objects, numbers or pictures are arranged into columns and rows) can help to develop understanding around this. Here’s what it might look like to build this knowledge from EYFS to Year 6.

EYFS: Grouping objects in different ways

Exploring how the same toys and objects can be grouped is an early precursor to thinking about the distributive law of multiplication.

Allow pupils to sort toys and other objects in any way they like. This also introduces them to equal and unequal sharing and grouping.

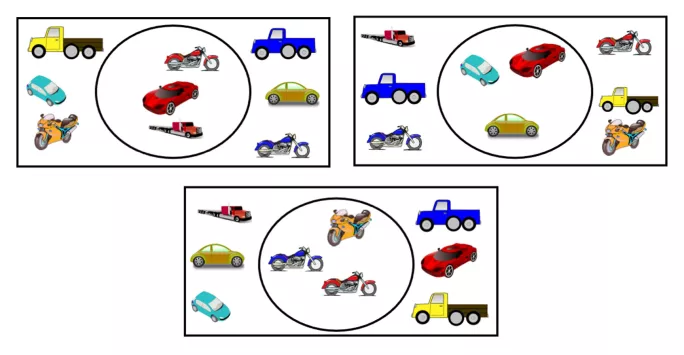
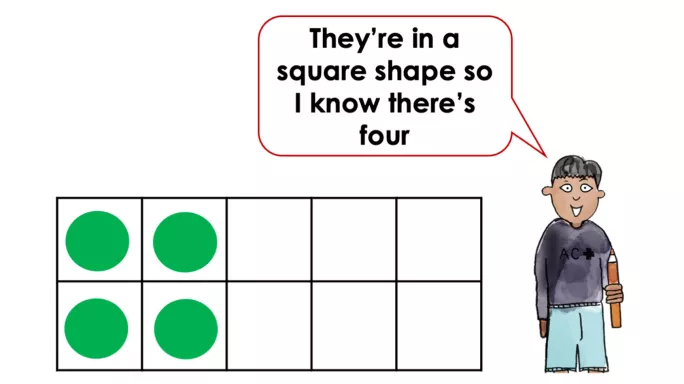
Formal arrays can then be introduced on fives frames, tens frames and dot arrangements, which encourage pupils’ number sense skill of subitising (recognising small quantities without counting them all).

Year 1: Equal and unequal groups

Arrays are explicitly referred to in the Year 1 national curriculum objectives, and it’s all about exploring them to develop the concept of equal groups in multiplication.

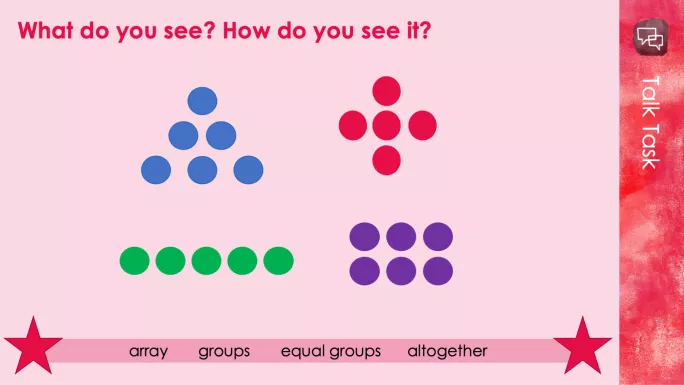
Seeing non-examples of this - via unequal groupings in different shapes - helps to develop understanding alongside describing and creating equally grouped rectangular arrays.

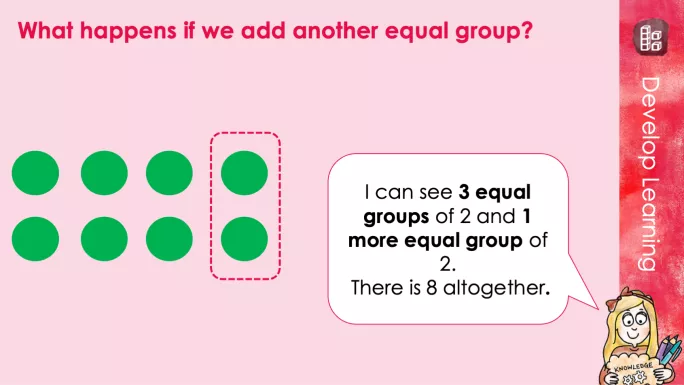
Lots of varied experience will help pupils to see and generalise that rectangular arrays always create equal groups, which can lead to early informal thinking about the distributive law, with questions such as “What happens if we add another equal group?” and “What happens if we remove a group?”.

Pupils can see 8 = 3 x 2 + 1 x 2 without even using the multiplication symbol introduced in Year 2.

Year 2: Commutativity and connecting multiplication to division

Arrays also pop up in the Year 2 national curriculum, with pupils tasked with constructing their own arrays, focusing on the number of equal parts and the value of each.

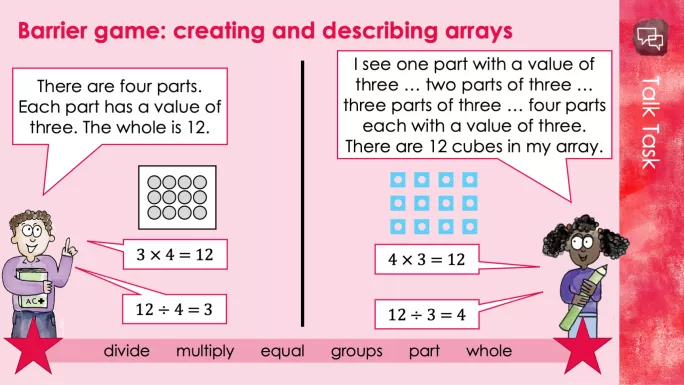
Through activities such as this paired barrier game, pupils can create and describe arrays, including how one array can be used to represent many different multiplication and division equations. The power of the array here is to explore commutativity, that we can see four equal groups of three but we can also see three equal groups of four. The whole is always 12.

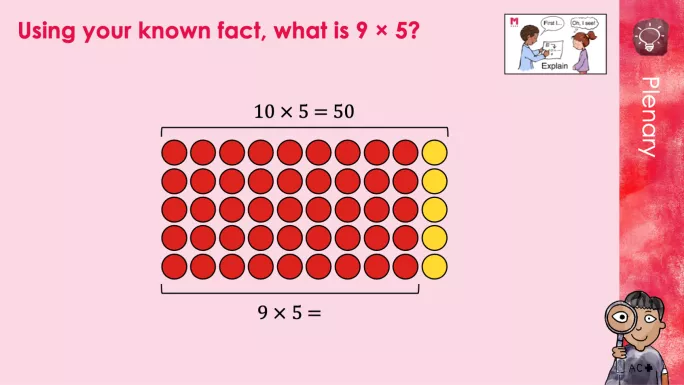
Splitting arrays in different ways and describing what they can see (“I can see 9 groups of 5 and 1 group of 5”) can support pupils to develop fluency within times tables.

Year 3: Deriving new facts from known facts

In Year 3, pupils apply the distributive law to multiply two- or three-digit numbers by one digit. They will have already explored what happens if you add a group or remove a group to an array in the times tables that they know. But how about questions such as 16 x 4 = ?.

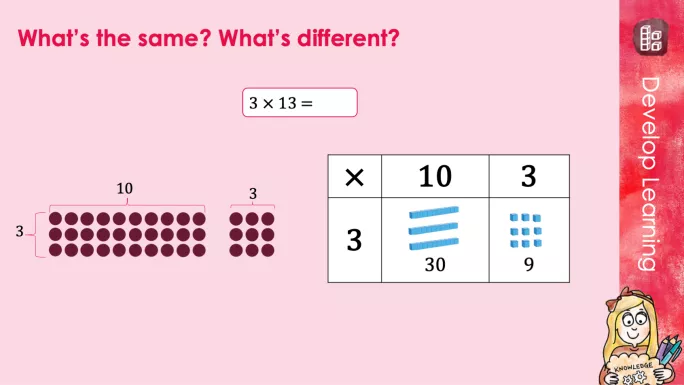
The array is a powerful representation to use here as it exposes the structure of the distributive law when used to derive facts beyond known times tables. The array can be partitioned to produce two smaller arrays, with number facts pupils can easily recall, for example: 3 x 13 = 3 x 10 + 3 x 3.

By splitting arrays in different ways, we are encouraging children to get into the habit of examining the numbers involved in a calculation and working flexibly to solve it.

Year 4: Arrays into area models

By the time pupils get to Year 4, most will have already used the distributive law informally, but this is the first time it’s explicitly referenced in the national curriculum.

When it comes to mentally multiplying larger numbers, as the factors involved become larger, the transitions from arrays of dots (or Dienes blocks) to area models become appropriate.

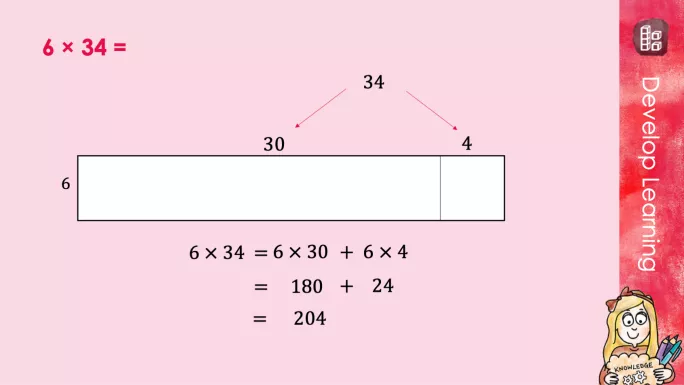
The area model provides a meaningful illustration of how partitioning can be used when multiplying larger numbers, while offering a clear visual of the magnitude of the numbers involved.

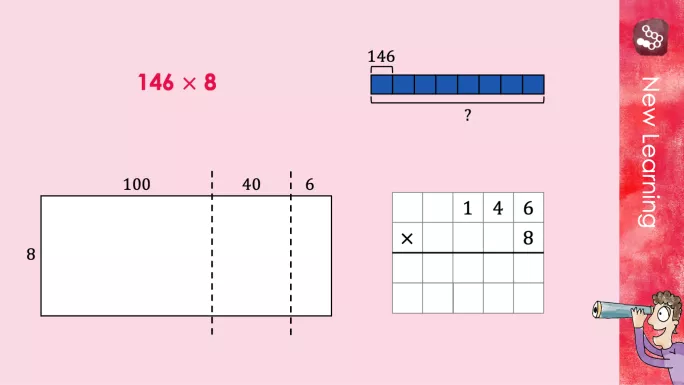
Formal methods can often be abstract concepts for pupils to get their heads around. See how the area model exposes the mathematical structure of the formal method of short multiplication. Applying the distributive law brings sense to this abstract method: 146 x 8 = 1,168 = 100 x 8 + 40 x 8 + 6 x 8.

Year 5: Making sense of long multiplication

The formal written method of long multiplication is introduced in Year 5.

The area model continues to support pupils in understanding how this abstract procedure works, based on the distributive law, for example 124 x 6 + 124 x 20 = 124 x 26.

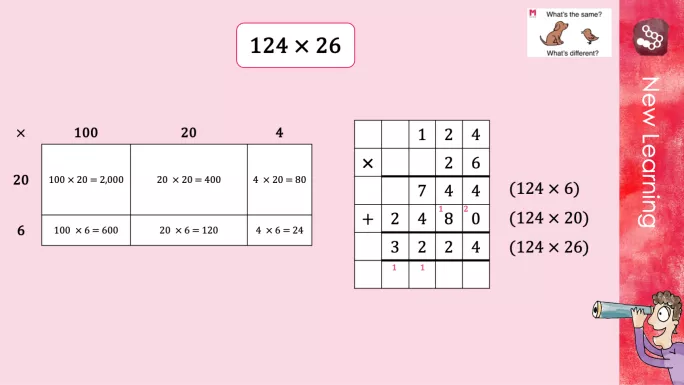
Look at the area model and how the calculations correspond with the steps in the long multiplication procedure. 124 x 6 is seen in the bottom row of the area model and 124 x 20 is seen in the top row of the area model.

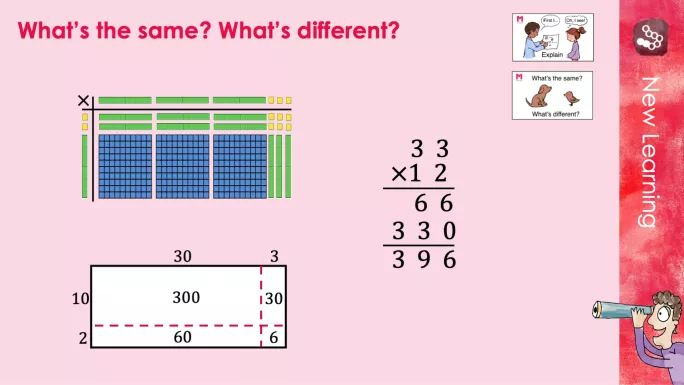
Both the Dienes representation and the area model highlight steps of the long multiplication method. The parts 6 + 60 and 30 + 300 are visually represented.

Year 6: How has the power of the array unlocked Sats success?

Let’s return to the Sats question at the beginning.

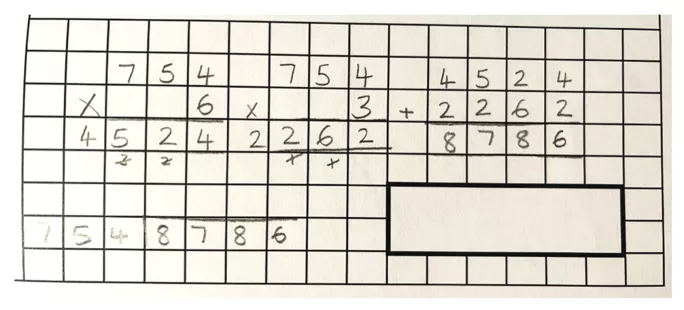
It turns out that, to solve it, there’s no need to use different formal written methods (repeated short multiplication, followed by formal addition, followed by long division).

Using their experience with multiple representations and conceptual understanding of multiplicative structure - specifically the distributive law, pupils can see that there are nine groups of 754.

6 groups of 754 + 3 groups of 754 = nine groups of 754 altogether.

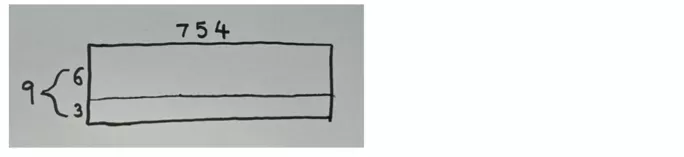
If they’ve had a coherent and connected journey from EYFS to Year 6, they may not even need to draw an area model.

From all the exploration of building and splitting arrays flexibly in lessons, they should have built up a good visual model to be able to answer this question successfully. And hopefully, they’ve enjoyed tackling an unfamiliar problem along the way, too.

Laura Tyler is head of Mathematics Mastery Primary

For the latest research, pedagogy and practical classroom advice delivered directly to your inbox every week, sign up to our Teaching Essentials newsletter